Lieu : Sorbonne Université, Jussieu / Collège de France
Référent : Nicolas Treps : nicolas.treps@lkb.upmc.fr
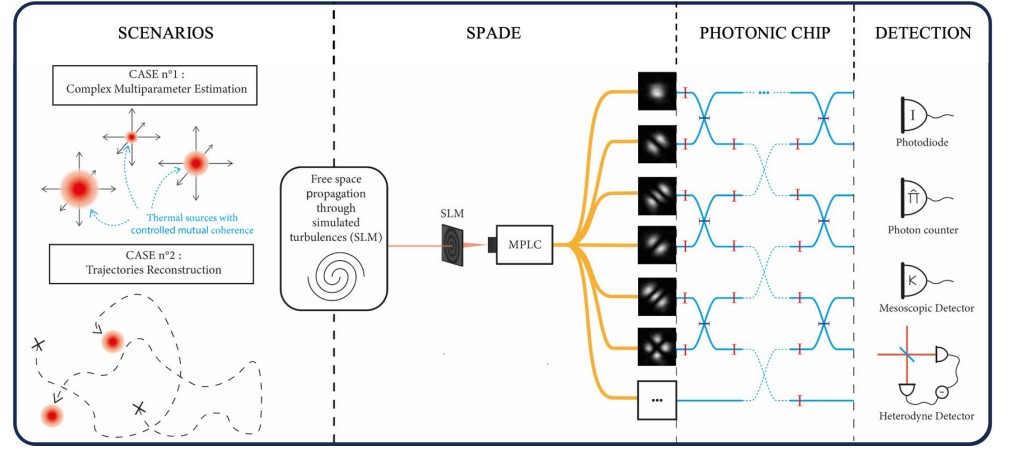
It was long believed that the ultimate resolution limit in imaging was dictated by the Rayleigh criterion, which states that two point sources are indistinguishable when their images overlap excessively. This diffraction limit, often considered a fundamental barrier in conventional imaging systems, posed a significant challenge for resolving closely spaced objects. However, recent advances in quantum metrology have revealed that the Rayleigh limit is not a fundamental boundary [1]. Employing non-conventional imaging techniques, inspired by quantum metrology, it is possible to achieve super-resolution imaging, surpassing the classical resolution limits [2,3]. One such approach is pursued in the PESto experiment at LKB, where Spatial Mode Demultiplexing (SPADE) is used. The light from two point sources is demultiplexed into a basis of Hermite-Gaussian spatial modes. Detecting and counting photons in each spatial mode of the multimode light, the distance between the two point sources is estimated with a precision approaching the quantum limit [4], order of magnitudes better than the Raileigh limit.
In practical imaging scenarios, multiple parameters must often be estimated simultaneously, making the problem more complex [5]. Notably, the SPADE technique is only quantum-optimal when only one parameter is to be estimated, and the others, such as the centroid of the source distribution, the relative intensity between the sources 0r even the number of sources, are known. This PhD project aims to extend the capabilities of SPADE to more realistic scenarios, incorporating multi-parameter estimation, low-flux detection down to the single photon level, and the effects of environmental factors such as optical turbulence. Addressing these complexities requires the integration of machine-learning techniques to optimize the choice of spatial modes, extract multiple parameters from the data efficiently, and ensure robustness against experimental imperfections. Additionally, in scenarios involving dynamic or moving sources—where only limited information can be gathered in real-time—a Bayesian approach to estimation will be explored to track the sources effectively.
This research will focus on advancing super-resolution imaging in realistic conditions, providing solutions to the challenges of multi-parameter estimation and developing methods to handle experimental imperfections and source motion. By working on both experiment and theory, leveraging estimation theory -classical and quantum-, machine learning and Bayesian techniques, the goal is to achieve unprecedented imaging precision and pave the way to a new paradigm in imaging.
[1] Tsang, M., Nair, R., & Lu, X. M. (2016). Quantum theory of superresolution for two incoherent optical point sources. Physical Review X, 6(3), 031033.
[2] Gessner, M., Treps, N., & Fabre, C. (2023). Estimation of a parameter encoded in the modal structure of a light beam: a quantum theory. Optica, 10(8), 996-999.
[3] Sorelli, M. Gessner, M. Walschaers, and N. Treps, Quantum limits for resolving Gaussian sources, Phys. Rev. Research 4, L032022 (2022).
[4] Rouvière, C., Barral, D., Grateau, A., Karuseichyk, I., Sorelli, G., Walschaers, M., & Treps, N. (2024). Ultra-sensitive separation estimation of optical sources. Optica, 11(2), 166-170.
[5] Řehaček, J., Hradil, Z., Stoklasa, B., Paúr, M., Grover, J., Krzic, A., & Sánchez-Soto, L. L. (2017). Multiparameter quantum metrology of incoherent point sources: towards realistic superresolution. Physical Review A, 96(6), 062107.
[6] C. Fabre and N. Treps, Modes and States in Quantum Optics, Rev. Mod. Phys. 92, 035005 (2020).

